Remember that we can check our answer by putting it into ALL of the original equations:

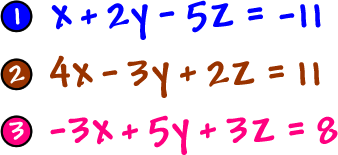
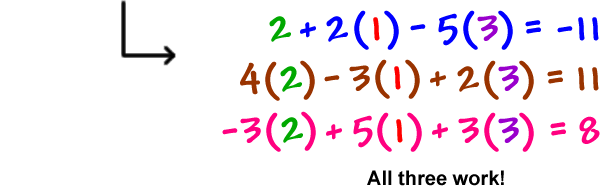
YOUR TURN:

Do the first problem, but start by ditching the x...  You'd better get the same answer I did!

Now, do the problem again, but start by ditching the z:

Here's another one for you:

* Remember to ditch the easiest guy!