Let's graph the inverse functions we had in the last lesson on the same graph and see what happens:
 

and

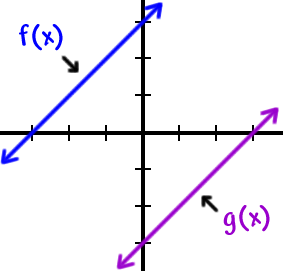
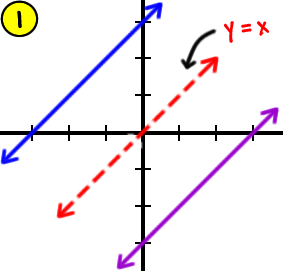
There are two big things I want you to notice:

They are mirror images over the line y = x. (In other words, they are symmetric with respect to the line y = x.)

 

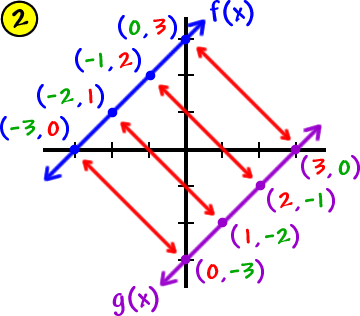Notice that every point on f(x) has a reversed partner on g(x). (0, 3) has (3, 0) as a partner and so on.

So, just remember this: