123
of 3

Solving Absolute Value Inequalties with Greater Than

Let's solve this guy:

| 2x + 1 | > 3


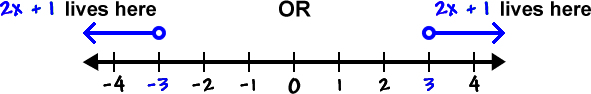
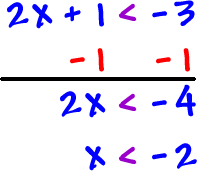a number line indicating 2x + 1 lives below -3 or 2x + 1 lives above 3


 

| 2x + 1 | < 3 ... subtract 1 from both sides ... 2x < -4 ... x < -2

or

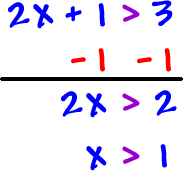 | 2x + 1 | > 3 ... subtract 1 from both sides ... 2x > 2 ... x > 1

 

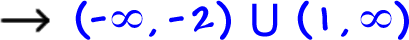interval notation (- infinity , -2 ) U ( 1 , infinity )

 

TRY IT:

Solve

| x - 2 | is greater than or equal to 4

 

Solve

2 | 3x + 1 | > 8

So... what about this guy?

| x + 5 | > -2

Think about what it's asking!

| x + 5 | > -2
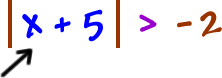
When is a distance greater than -2?

Always, silly!

We can't even set this guy up to TRY to solve him.

The answer is

( - infinity , infinity )