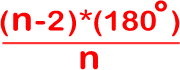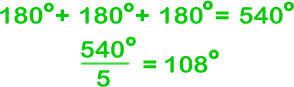METHOD 1:
Let's divide some regular polygons into triangles by connecting one vertex to all of the others...
|
|
| ||
A square has 4 sides and we made 2 triangles. | A pentagon has 5 sides and we made 3 triangles. | A hexagon has 6 sides and we made 4 triangles. |
Do you see the pattern?
A heptagon has 7 sides... so we'd be able to make 5 triangles.
If we had polygon with n sides... we'd be able to make (n - 2) triangles.
Let's start with the square... We made 2 triangles. Notice that all of the interior angles of the 2 triangles make up the interior angles of the square.
The sum of the 2 triangle's angles is 
There are 4 equal angles in a square,
so 
gives us that one angle of a square is 
!
Just what we expected.
Now for the pentagon.
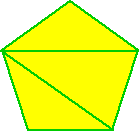 | We made 3 triangles.
|
So the interior angles of a regular pentagon are each 108 degrees.
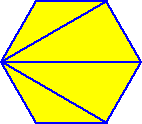
Can you figure out the hexagon?
 |  |
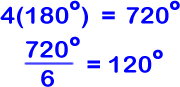How about a 100-gon? (That's a regular polygon with 100 sides.) There would be 98 triangles...
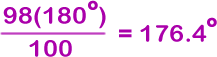
So, in general, the measure of an interior angle of a regular n-gon is
