12
of 2

Increasing and Decreasing

If Pierre is going downhill, then the graph is decreasing:

on the interval ( -3 , 1 ) , Pierre is sliding downhill ( Wheeee! ) ... therefore, the graph is decreasing

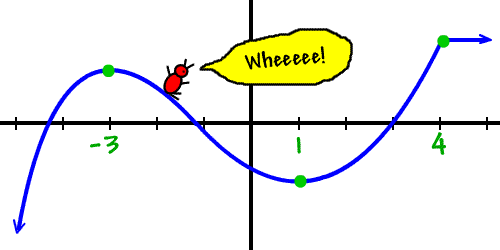
So, our graph is decreasing on

-3 , 1

Notice that I'm not using the endpoints on these:

Like

  [ -3 , 1 ] ... closed interval

Think about it...  When Pierre is standing right ON x = -3 ...

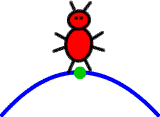
Piere is standing on x = -3 ... the graph is not increasing or decreasing

he's not going uphill or downhill. He's just standing there!

What if Pierre is walking on   ( 4 , infinity )  ?

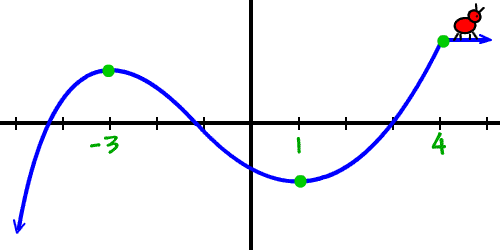
 on the interval ( 4 , infinity ) , Pierre is walking straight ... the graph is not increasing or decreasing

That line is horizontal (slope of 0). He's not going uphill or downhill, so the graph is not increasing or decreasing there.

TRY IT:

For the following graph, list the intervals where the graph is increasing and decreasing:

list the intervals where the graph is increasing and decreasing

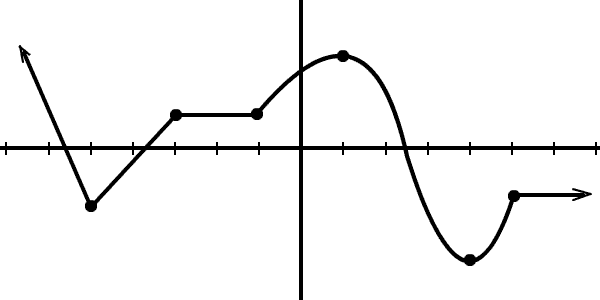
*Remember to answer in interval notation using only x values (no y values allowed)!